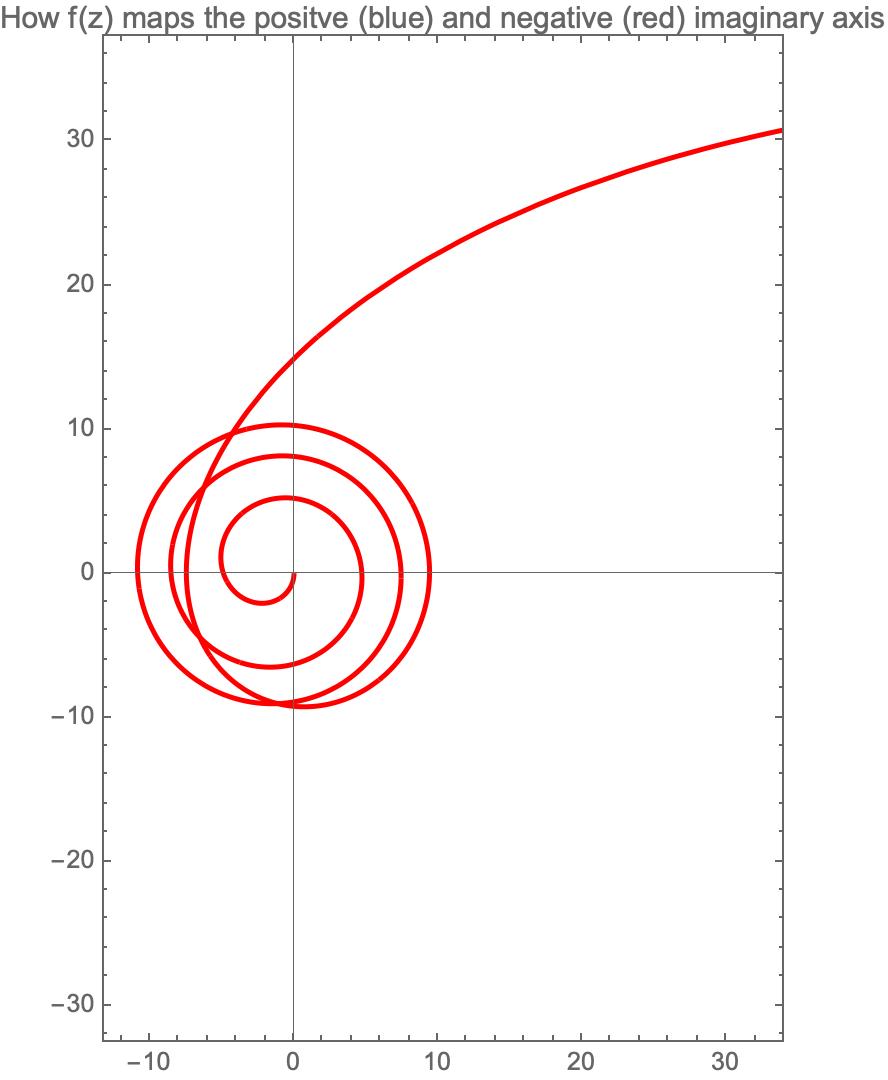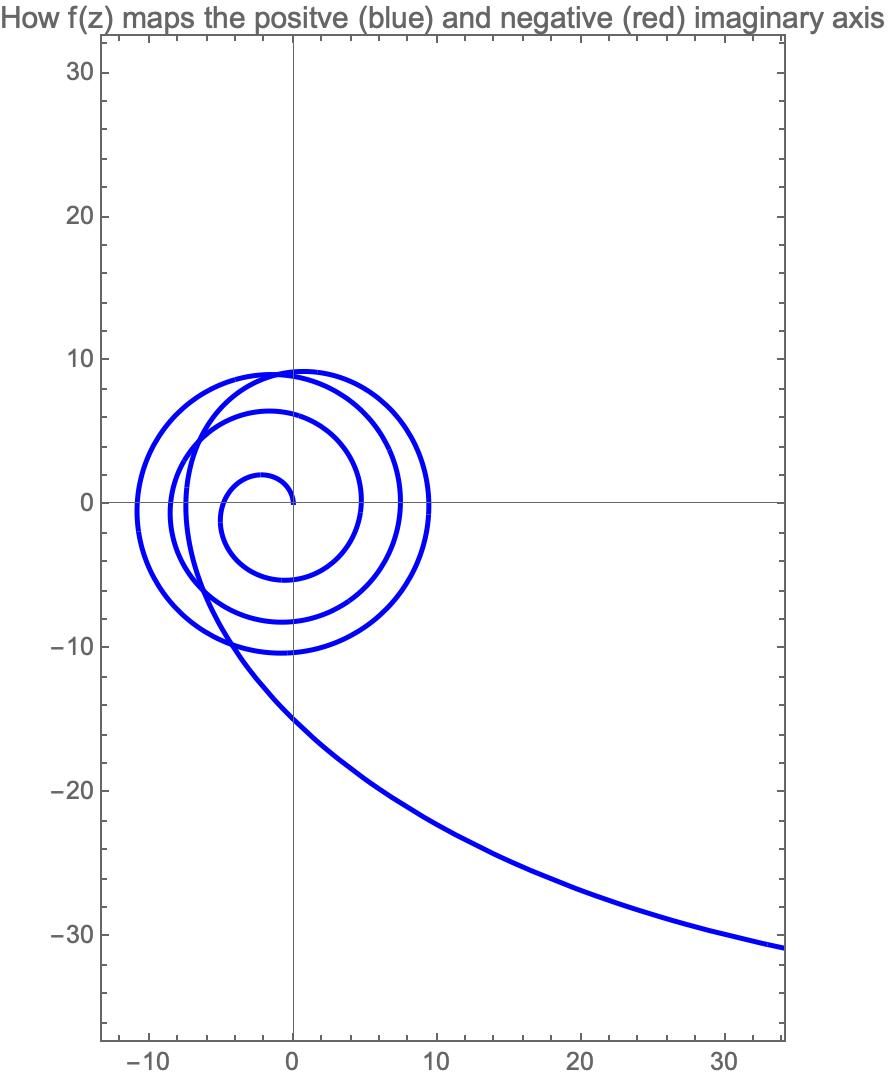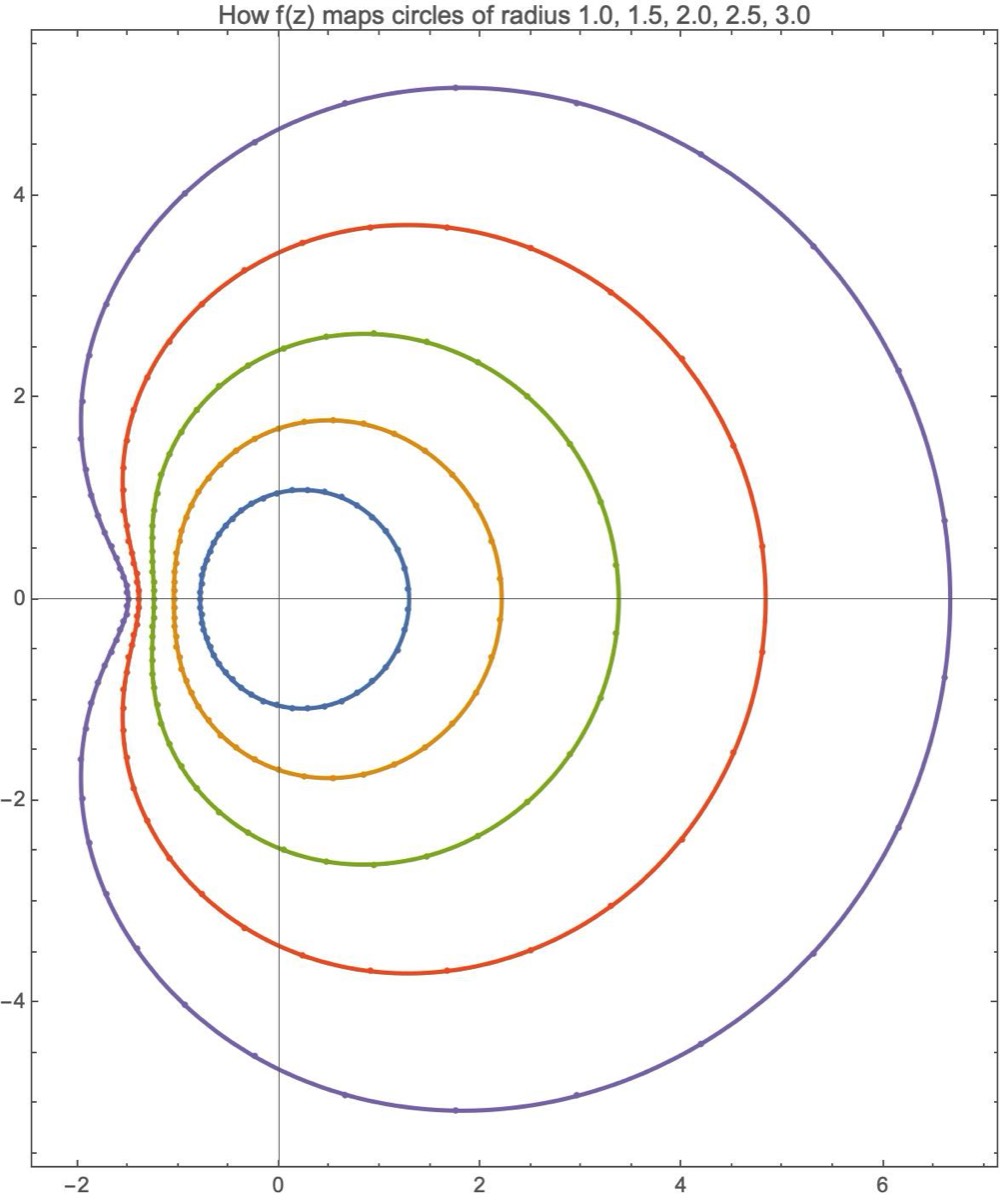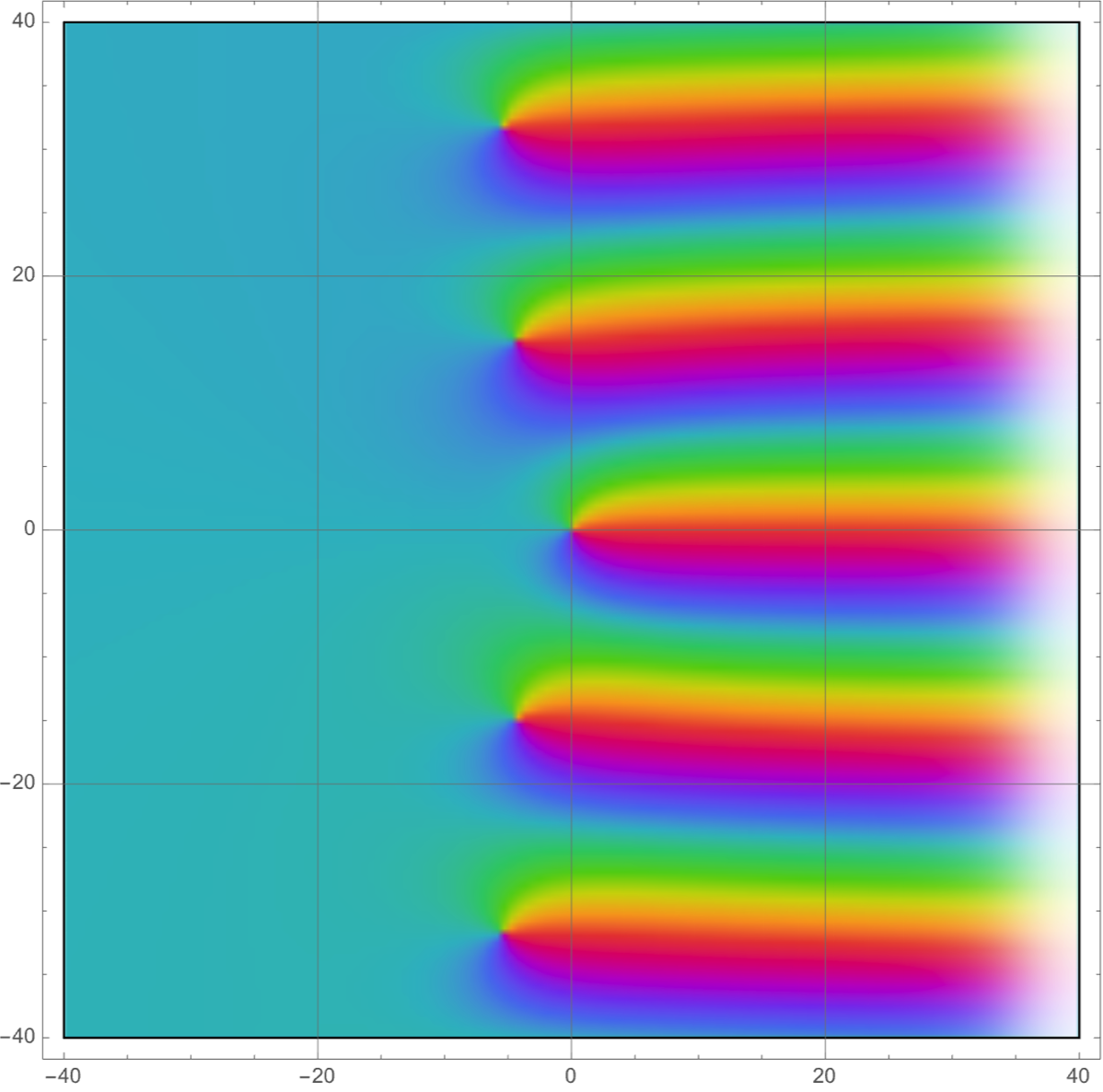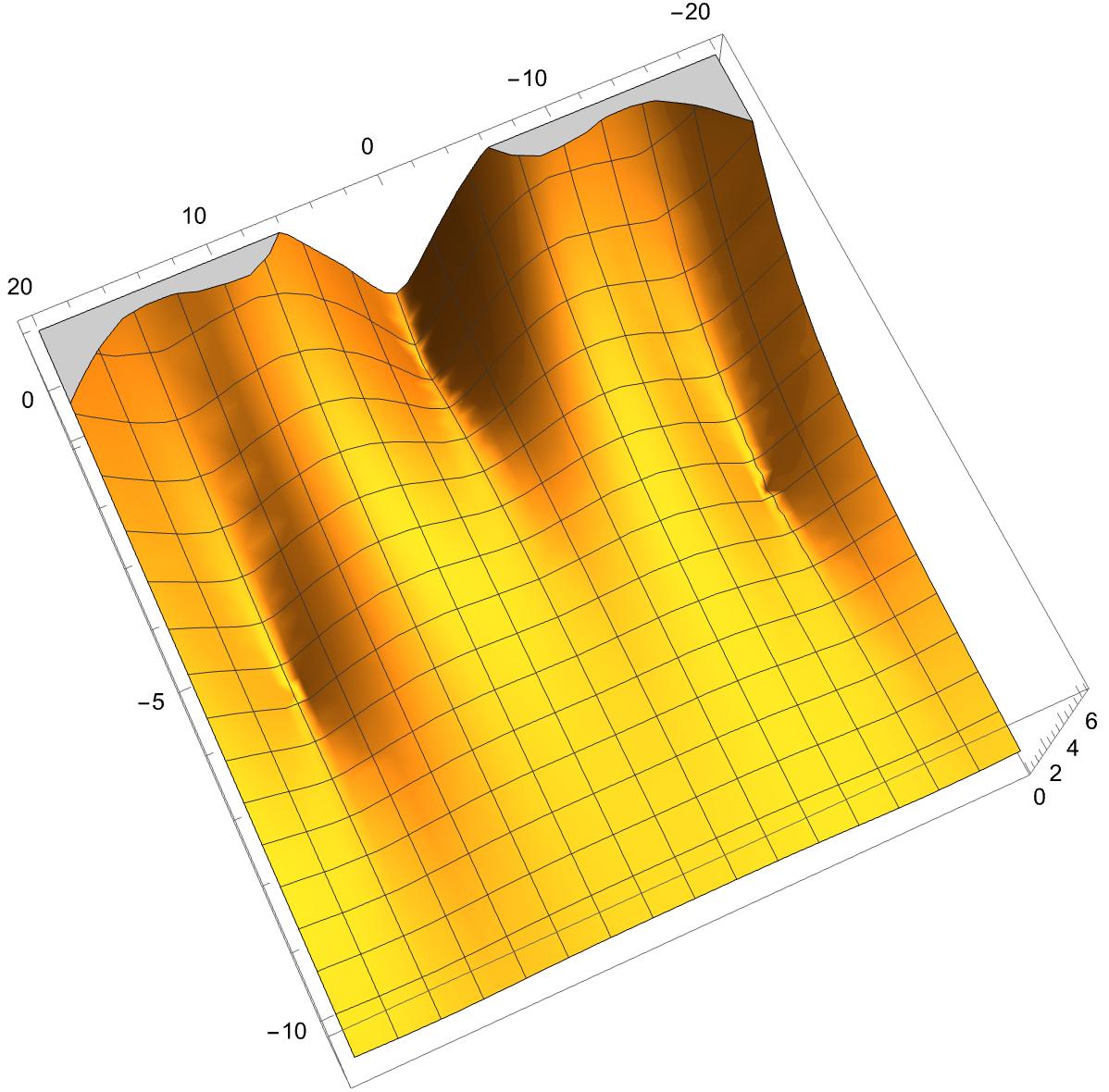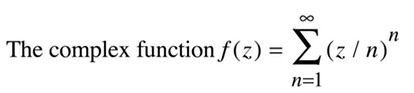
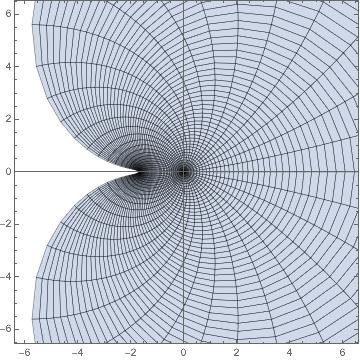
I find this function interesting. The power series coefficients, (1/n)n decrease quite rapidly, faster than 1/n!. By the root test, for example, the infinite series converges everywhere (infinite radius of convergence), so the function is entire (holomorphic in the entire complex plane). To the best of my knowledge, it is unproven whether the value f(1)=1.291285997...is transcendental or even irrational. See Weisstein, Eric W. "Sophomore's Dream." From MathWorld – A Wolfram Web Resource. Sophomore's Dream. The plot above is a parametric plot of the real and imaginary parts of f(z), (Re(f(z)), Im(f(z))), for Abs(z) between 0 and 5 and Arg(z) from 0 to 2π. It can be hard to see a pattern here, so another approach is to decompose it into separate plots. The first two plots below show how f(z) maps the imaginary axis (i.e., points of the form 0+yi). It maps the imaginary axis into an expanding spiral shape. Increasing negative values of y map to a clockwise spiral (red), and positive values map to a counterclockwise spiral (blue). The third plot shows that f(z) maps circles of small radius into oval shapes, where the positive real side expands, and the negative real side is pinched in. The fourth and fifth plots of the absolute value of f(z) suggest there are values besides zero where f(z)=0. For example, the absolute value of f(-4.30478021156984 ± 14.9450782537071*i]) is less than 10-12.